Andrzej Boguslawski
Częstochowa University of Technology, Poland
Title: LES predictions of self-sustained oscillations in round free jets
Biography
Biography: Andrzej Boguslawski
Abstract
The paper is devoted to recent advancements in numerical simulations of round free jets, which are in common use in many technical applications and which have been widely investigated experimentally and numerically. The attention is attached to conditions under which self-sustained global modes can be triggered in homogeneous and variable density round jets. Global modes result from a phenomenon of absolute instability in low density jets. This phenomenon predicted by linear stability theory was confirmed experimentally in hot air-jet and in air-helium jets. However, as shown recently, self-sustained global oscillations can be released in homogenous density convectively unstable jets provided that a shear layer at the nozzle exit is sufficiently thin. In such a case, a rapid growth of the Kelvin-Helmholtz mode induces a back-flow leading to self-sustained resonant jet oscillations. It was demonstrated by Boguslawski et al. (2013) and Wawrzak et al. (2015) that such a self-excited mechanism requires a low turbulence level and thin shear layer thickness at the nozzle exit characterized by the momentum thickness R/θ = 25 (θ - momentum thickness, R-radius of the nozzle). In Figure 1 the Q-parameter Q = √2 (|Ω|2 - |S|2) (Ω, S- vorticity and strain rate tensors) exhibits toroidal structures resulting from the jet instability. The results are presented for two shear layer thicknesses with R/θ= 20 and R/θ = 28. In the latter case, when the critical thickness is exceeded, the formation of strong vortex structures is observed near the inlet plane, which consequently pair at a distance x/D ≈ 2.9. To the authors’ knowledge, such a self-sustained regime in the homogeneous- density jet is a new phenomenon not reported in the literature so far.
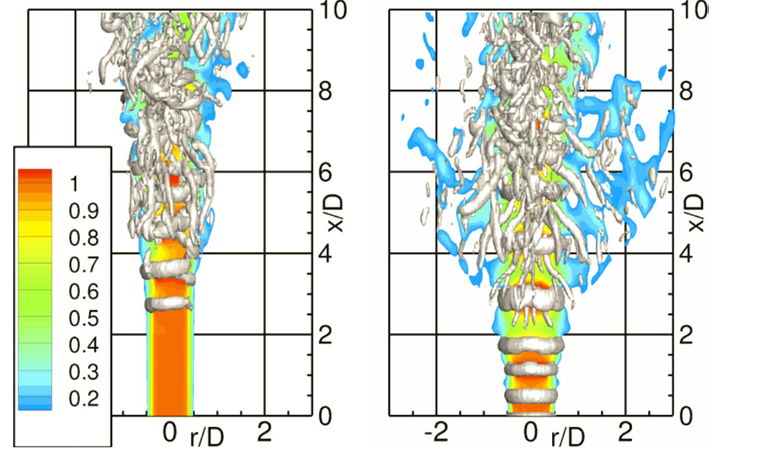
Figure 1: Iso-surfaces of Q - parameter and contours of the axial velocity in the main cross-section. R/ θ =20 -left figure, R/ θ =28 -right figure.
Recent Publications
1.Boguslawski A, Tyliszczak A, Drobniak S and Asendrych D (2013) Self-sustained oscillations in homogeneous-density round jet. Journal of Turbulence 14(4):25–52.
2.Wawrzak K, Boguslawski A and Tyliszczak A (2015) LES predictions of self-sustained oscillations in homogeneous density round free jet. Flow, Turbulence and Combustion 95(2-3):437–459.
3. Boguslawski A, Tyliszczak A, Wawrzak A (2016) LES predictions of unstable round hot jet. Physics of Fluids doi: http://dx.doi.org/10.1063/1.4941656.
4. Monkewitz P A, Bechert D W, Barsikow B and Lehmann B (1990) Self-excited oscillations and mixing in a heated round jet, Journal of Fluid Mechanics 213:611–639.
5. Hallberg M P, Strykowski P J (2006) On the universality of global modes in low-density axisymmetric jets. Journal of Fluid Mechanics 569:493-507
