Fluid Dynamics & Aerodynamics

Biography

Biography: Xesús Nogueira

Abstract

The development of numerical methods for the simulation of problems involving highly complex geometries, which are frequent in many engineering problems, remains a very active research field in computational fluid dynamics (CFD). In the simulation of turbulent flows, for example, high-accurate numerical techniques are required to account for the fine scales of the flow. In these kinds of problems, the construction of a good quality mesh is one of the most time-consuming procedures. Another example is Computational Aeroacoustics (CAA), where the low magnitude of acoustic waves and the wide range of frequencies involved, makes mandatory the use of very accurate numerical schemes. Nowadays, the standard numerical techniques in CAA are mainly grid-based methods. However, the use of Lagrangian methods in the context of CAA may be interesting for problems involving deformable or moving boundaries in the propagation media or multiphase flows, such as combustion noise, sound propagation through multiphase flows or bubble acoustics. Unfortunately, there are very few Lagrangian numerical methods to solve CAA problems in the literature. In this work, we propose a new high-accurate, stable and low- dissipative meshless method based on a Galerkin discretization of a set of conservation equations on an ALE approach, using moving least squares as weight functions for the Galerkin discretization. The stability of the scheme is achieved by the recent a posteriori multi-dimensional optimal order detection paradigm. We apply the numerical scheme to some test cases including CAA (Figure 1) and compressible flows. The proposed numerical method can be seen as a general formulation which includes some well-known meshfree methods. The promising results obtained shown that the proposed scheme can be applied to problems with engineering interest.

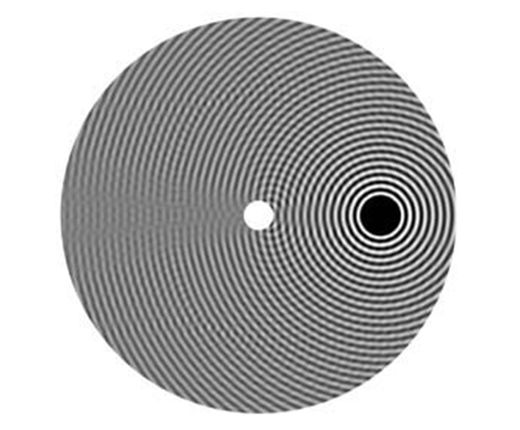
Figure 1: Time-periodic Gaussian source scattering by a circular cylinder computed with the proposed scheme.

Recent Publications

1.  Y O Zhang, T Zhang, H Ouyang, T Y Li (2016) Efficient SPH simulation of time-domain acoustic wave propagation. Engineering Analysis with Boundary Elements 62:112-122.

2.   S Clain, S Diot, R Loubère (2011) A high-order finite volume method for systems of conservation laws- Multidimensional Optimal Order Detection (MOOD). Journal of Computational Physics 230:4028-4050.

3. J P Vila (1999) On particle weighted methods and smooth particle hydrodynamics. Mathematical Models and Methods in Applied Sciences 9(2):161-209.

4.  X Nogueira, L Ramírez, S Clain, R Loubère, L Cueto-Felgueroso and I Colominas (2016) High-accurate SPH method with multidimensional optimal order detection limiting. Computer Methods in Applied Mechanics and Engineering 310:134-155.

5. E Gaburov and K Nitadori (2011) Astrophysical weighted particle magnetohydrodynamics. Monthly Notices of the Royal Astronomical Society 414:129-154.